User Guide
Accuracy Analysis
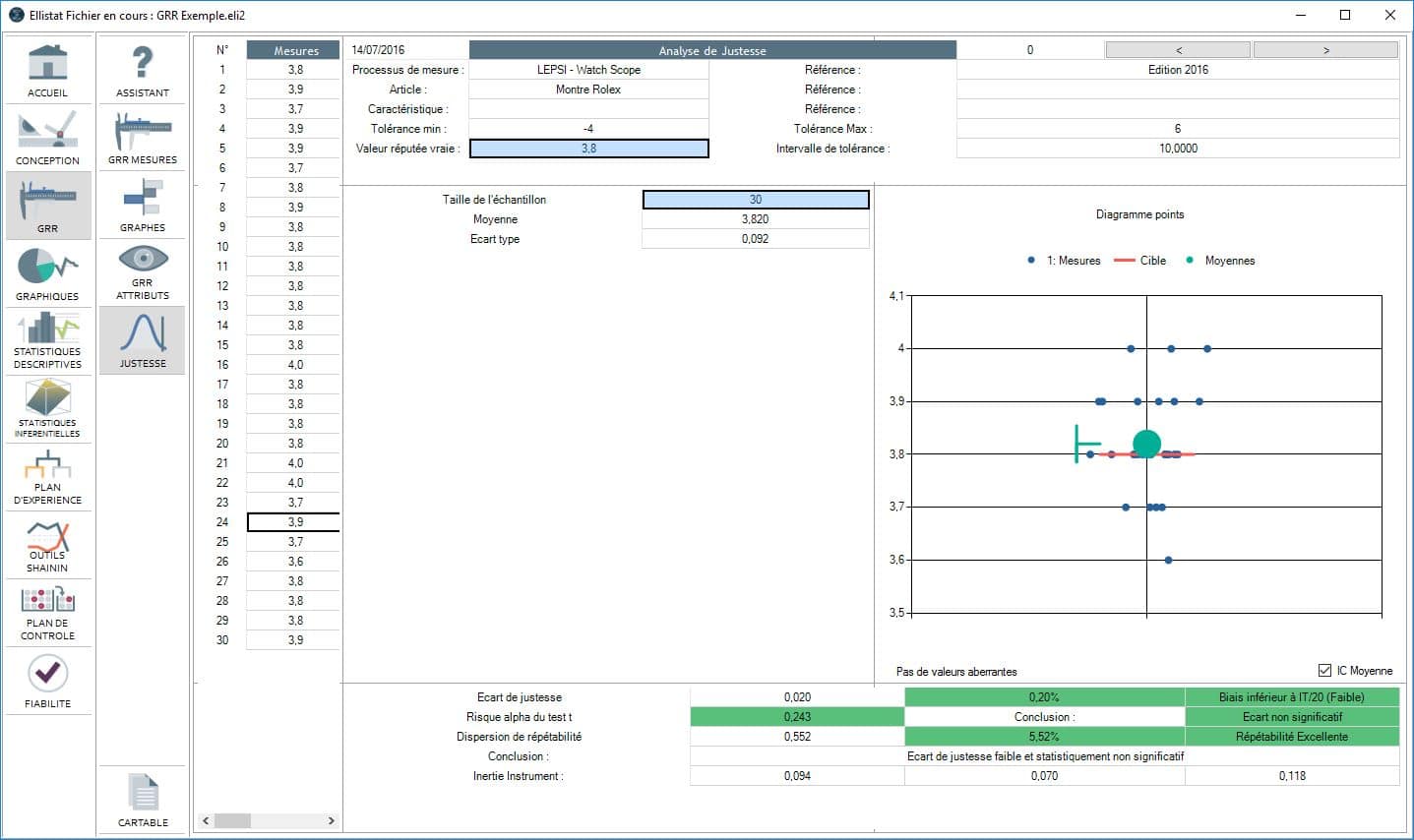
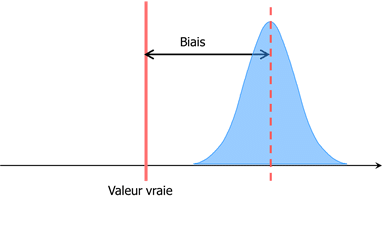
The accuracy analysis of a measurement instrument makes it possible to verify that the average measurement does not differ from the true value of the measurand. We call any difference a measurement bias. If this difference is significant, it must be corrected.
To calculate the measurement bias, 30 successive measurements are carried out on a part for which the true value is known (a benchmark, for example).
Next the mean of these measurements is compared with the part’s true value. Suppose that the true value of the part is 7.702.

The bias is calculated by:
The bias is significant if it is higher than IT/20.
Bias Significance
When calculating the bias of a measurement process by taking the average of a series of measurements, the probability of calculating the exact null bias is close to 0. It is thus advisable to question the significance of the bias. I.e., the calculated bias is significantly different than 0 in the statistical sense of the term.
For this, the calculation of the bias is represented by its standard deviation number.
The value limit of t is calculated by using the Student table for n-1 degrees of freedom and an alpha bilateral risk of 5%, or an alpha unilateral risk of 2.5%.
For example, in the preceding example of 30 consecutive measurements
The bias is significant if t>tlimit.

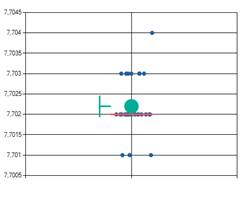
The graph above makes graphic representation of the bias and the significance of the bias possible.
- The blue points represent measurements.
- The green point represents the calculated average and the green line on the left represent the confidence interval of the average.
- The red line represents the true value of the measurand. If this is located inside the confidence interval, the bias is not significant.
- The contrary is true, that if this line is outside of the confidence interval then the bias is significant.